Overview
The free energy change for a reaction that occurs under the standard conditions of 1 bar pressure and at 298 K is called the standard free energy change. Since free energy is a state function, its value depends only on the conditions of the initial and final states of the system. A convenient and common approach to the calculation of free energy changes for physical and chemical reactions is by use of widely available compilations of standard state thermodynamic data. One method involves the use of standard enthalpies and entropies to compute standard free energy changes, ΔG°, according to the following relation.
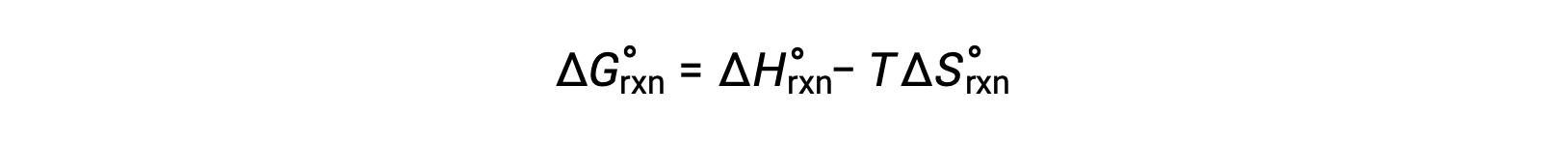
The standard free energy change for a reaction may also be calculated from the standard free energy of formation ΔGf° values of the reactants and products involved in the reaction. The standard free energy of formation is the free energy change that accompanies the formation of one mole of a substance from its elements in their standard states. Similar to the standard enthalpy of formation, ΔGf° is by definition zero for elemental substances under standard state conditions. For the reaction
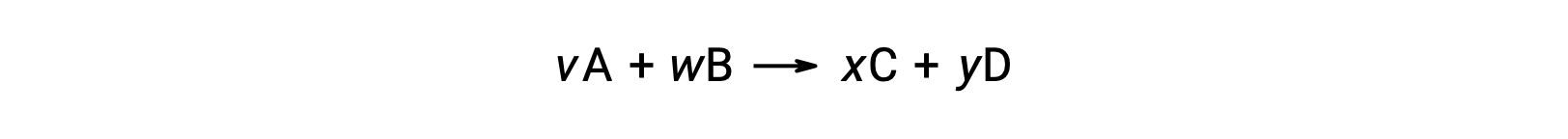
the standard free energy change at room temperature may be calculated as
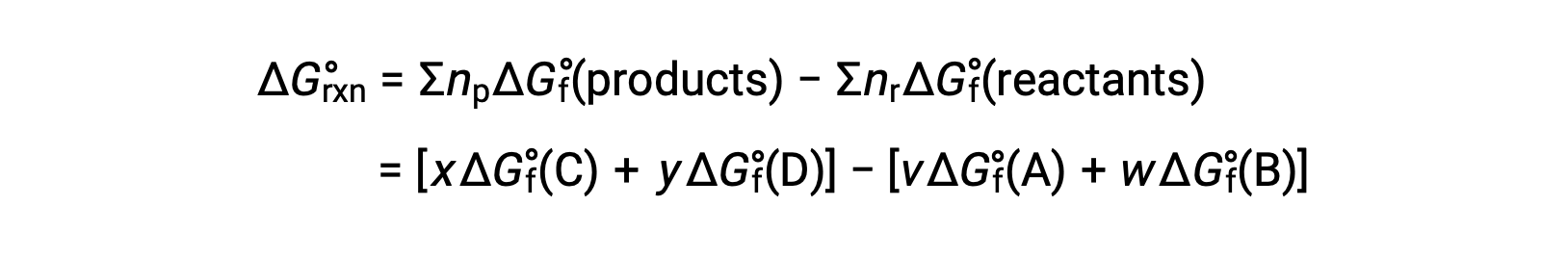
The use of free energies of formation to compute free energy changes for reactions as described above is possible because ΔG is a state function, and the approach is analogous to the use of Hess’ Law in computing enthalpy changes. Consider the vaporization of water as an example:
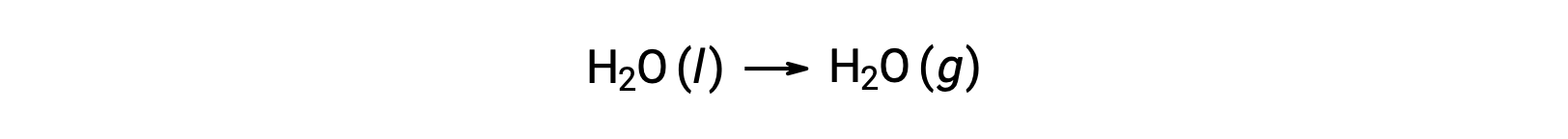
An equation representing this process may be derived by adding the formation reactions for the two phases of water (necessarily reversing the reaction for the liquid phase). The free energy change for the sum reaction is the sum of free energy changes for the two added reactions:
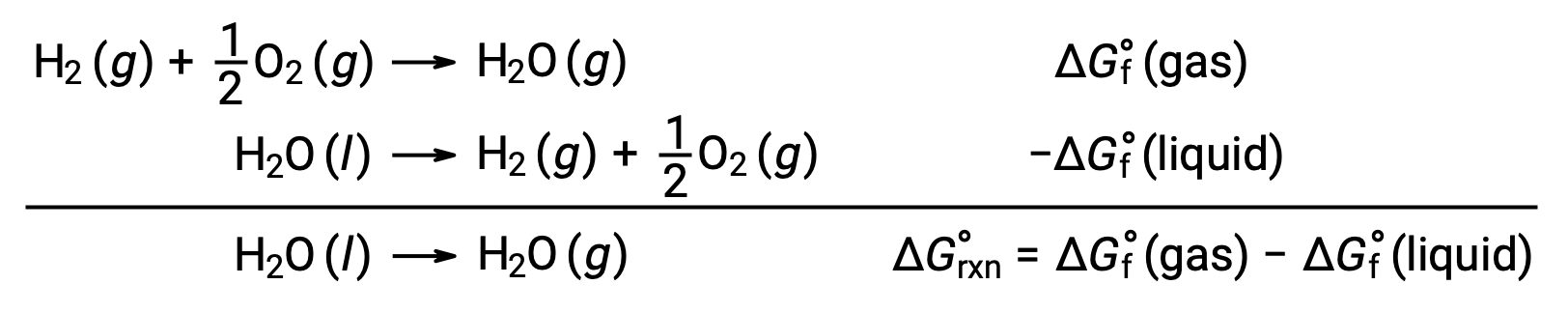
This approach may also be used in cases where a nonspontaneous reaction is enabled by coupling it to a spontaneous reaction. For example, the production of elemental zinc from zinc sulfide is thermodynamically unfavorable, as indicated by a positive value for ΔG°1:

The industrial process for the production of zinc from sulfidic ores involves coupling this decomposition reaction to the thermodynamically favorable oxidation of sulfur:

The coupled reaction exhibits a negative free energy change and is spontaneous:
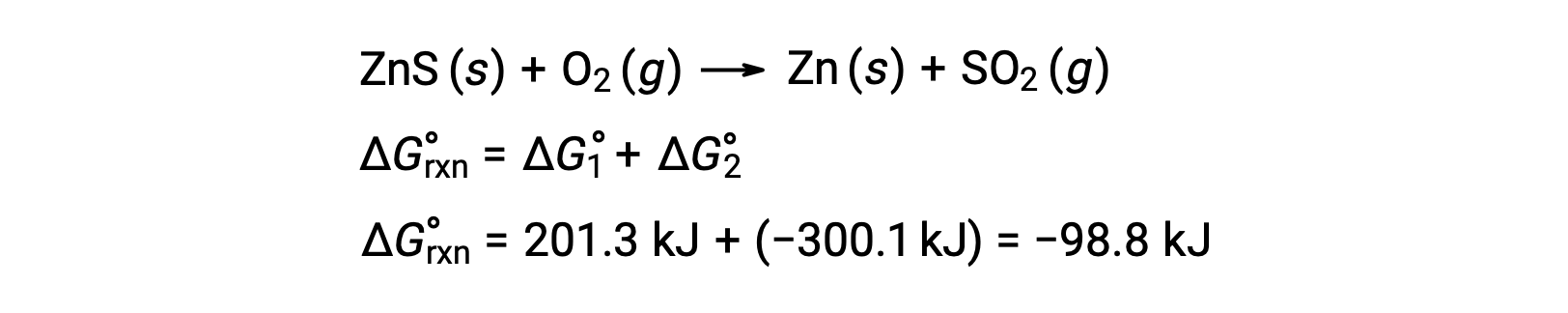
This process is typically carried out at elevated temperatures, so this result obtained using standard free energy values is just an estimate. The gist of the calculation, however, holds true.
This text is adapted from Openstax, Chemistry 2e, Chapter 16.4: Free Energy.
Procedure
The standard free energy change for a chemical reaction occurring under the standard condition can be calculated in one of three ways.
The first method uses the equation for the standard ΔG of the reaction. Consider the formation of calcium carbonate from calcium oxide and carbon dioxide.
The standard enthalpy change of the reaction can be calculated from the heats of formation of the compounds in the reaction, and the standard entropy change can be calculated from their standard molar entropies.
Using the values from the reference tables, the standard enthalpy change and the standard entropy change for the reaction can be determined.
Substituting these values into the equation for the change in standard free energy, the standard ΔG of the reaction at 298 K equals −130.7 × 103 J.
In the second method, the change in standard free energy of a reaction is calculated from the difference between the sum of the free energies of formation of the products multiplied by their stoichiometric coefficients and the sum of the free energies of formation of the reactants multiplied by their stoichiometric coefficients.
Take, for example, the reaction between hydrogen and chlorine gases to form two moles of hydrogen chloride gas.
Like the enthalpy of formation, the standard free energy of formation of a pure element is zero.
Thus, the free energies of formation of hydrogen and chlorine gases are zero, while that of hydrogen chloride gas is −95.3 kilojoules.
So, the free energy of this reaction equals 2 times the free energy of formation of hydrogen chloride gas, or −190.6 kilojoules.
Recall from Hess’s law that for a stepwise reaction, the net enthalpy change can be calculated from the sum of the enthalpies of each step. The third method calculates the net free energy change for a reaction in a similar manner.
Consider the combustion of zinc sulfide to produce metallic zinc and sulfur dioxide gas, which can be carried out in two steps with known standard free energies.
Adding the free energy changes of the reactions gives an overall ΔG of −98.8 kilojoules.
Note that step 1 is nonspontaneous. By coupling it with the spontaneous reaction in step 2, the net reaction has a negative ΔG and is therefore spontaneous.