Overview
Source: Laboratory of Dr. Andreas Züttel - Swiss Federal Laboratories for Materials Science and Technology
The ideal gas law describes the behavior of most common gases at near-ambient conditions and the tendency of all chemical matter in the dilute limit. It is a fundamental relationship between three measurable macroscopic system variables (pressure, temperature, and volume) and the number of molecules of gas in the system, and is therefore an essential link between the microscopic and the macroscopic universes.
The history of the ideal gas law dates to the middle of the 17th century when the relationship between the pressure and volume of air was found to be inversely proportional, an expression confirmed by Robert Boyle and which we now refer to as Boyle’s law (Equation 1).
P 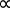 V-1 (Equation 1)
V-1 (Equation 1)
Unpublished work by Jacques Charles in the 1780s, which was extended to numerous gases and vapors by Joseph Louis Gay-Lussac and reported in 1802, established the directly proportional relationship between the absolute temperature and volume of a gas. This relationship is called Charles's law (Equation 2).
V 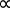 T (Equation 2)
T (Equation 2)
Guillaume Amontons is typically credited with first discovering the relationship between the temperature and pressure of air within a fixed volume at the turn of the 18th century. This law was also extended to numerous other gases by Joseph Louis Gay-Lussac at the beginning of the 19th century and is therefore either referred to as either Amontons’s law or Gay-Lussac's law, stated as shown in Equation 3.
P 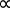 T (Equation 3)
T (Equation 3)
Together, these three relationships can be combined to give the relationship in Equation 4.
V 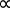 T (Equation 4)
T (Equation 4)
Finally, in 1811, it was proposed by Amedeo Avogadro that any two gases, held in the same volume and at the same temperature and pressure, contain the same number of molecules. This led to the conclusion that all gases may be described by a common constant, the ideal gas constant R, that is independent of the nature of the gas. This is known as the ideal gas law (Equation 5).1,2
PV 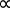 T (Equation 5)
T (Equation 5)
Principles
The ideal gas law, and therefore its characteristic constant R, can also be eloquently derived from first-principles theory in numerous ways, where the important simplifying assumptions are that the molecules have no inherent volume and do not interact. These assumptions are valid in the dilute matter limit, where the volume of empty space occupied by each molecule (e.g. ~10-23 L at ambient conditions) is much larger than the molecule itself (~10-26 L), and where interactions are improbable. It can therefore easily be demonstrated in a number of ways using common laboratory equipment at room temperature, and can be accurately measured using a variety of gases at pressures up to even 10 bar (Figure 1). However, the ideal gas law cannot accurately account for the properties of denser gases at near-ambient conditions (e.g., propane) or for condensation, phenomena that arise as a result of intermolecular interactions. For this reason, numerous more detailed equations of state have succeeded the ideal gas law in the years since its discovery, typically reducing to the ideal gas law in the dilute matter limit.1,2

Figure 1. Comparison of the ideal gas law density to various other common gases at 25 °C and between 0-100 bar.
In this tutorial, we will carefully measure the density of hydrogen gas at increased pressures and temperatures within a fixed volume by weighing a suspended solid sample of known volume: a precisely machined aluminum block. The change in weight of the sample is directly related to the change in fluid density, in which it is floating, by Archimedes’s principle. We will also demonstrate the shortcomings of using a less ideal gas (such as carbon dioxide) at high pressures. Finally, we will visually demonstrate and qualitatively confirm the ideal gas law by performing a simple benchtop experiment where the change in volume of the system due to hydrogen release by a hydrogen storage material is measured. Using any one of these experiments, we can determine the universal constant that describes the relationship between pressure, temperature, and volume for a given amount of gas – the ideal gas constant, R.
Procedure
1. Measuring the Volume of the Sample
- Clean the sample carefully and dry it.
- Fill a high-resolution graduated cylinder with enough distilled water to cover the sample. Note the initial volume
- Drop the sample into the water and note the volume change. This is the volume of the sample, V.
- Remove the sample and dry it. Note: alternatively, measured the side length(s) of the sample and calculate its volume using geometry.
2. Load the Sample in the Balance
- Hang the sample in the magnetic suspension balance.
- Install the pressure/temperature chamber around the sample.
- Evacuate the sample environment and refill with hydrogen gas, to 1 bar.
- Measure the sample weight at 1 bar and room temperature, w00.
3. Measure Sample Weight as a Function of Pressure at Room Temperature
- Increase or decrease the pressure in the sample environment to Pi0.
- Allow the sample environment to equilibrate.
- Measure the weight of the sample, wi0.
- Repeat 3.1-3.3 numerous times.
4. Measure Sample Weight as a Function of Pressure at Various Temperatures
- Set the temperature to Tj and allow it to equilibrate.
- Set the pressure of hydrogen gas to 1 bar.
- Measure the sample weight at 1 bar and Tj, w0j.
- Increase or decrease the pressure to Pij and allow it to equilibrate.
- Measure the weight of the sample, wij.
- Repeat 4.4-4.5 numerous times.
- Repeat 4.1-4.6 as desired.
5. Calculate the Ideal Gas Constant
- Tabulate the measured values {Tj, Pij, and wij} where P0j is always 1 bar and T0 is the measured room temperature.
- Calculate and tabulate the differences Δwij and ΔPij at each temperature Tj using Equation 6 and Equation 7.
Δwij = wij - w0j (Equation 6)
Δwij = Pij - P0j = Pij - 1 bar (Equation 7) - Calculate Rij for each measurement, and average over all values to determine the ideal gas constant, R. Alternatively, plot the product of ΔPij and V as a function of the product of Δwij (divided by the molecular weight, MW) and Tj, and perform a linear regression analysis to determine the slope, R. (Equations 8 and 9) For hydrogen, MW = 2.016 g/mol.
ΔP V = Δn RT (Equation 8)
 (Equation 9)
(Equation 9)
Results
The ideal gas law is a valid description of the actual gas properties of numerous common gases at conditions near ambient (Figure 1 inset) and is therefore useful in the context of many applications. The limitations of the ideal gas law in describing systems under conditions of high pressures or low temperatures can be explained by the increasing importance of molecular interactions and/or the finite size of the gas molecules contributing to the system’s properties. Therefore, gases with strong, attractive intermolecular interactions (arising from dipole-dipole interactions, including hydrogen bonding, ion-dipole interactions, or van der Waals interactions) will exhibit higher densities than the ideal gas. All gases will also have a repulsive component at high densities due to the fact that more than one molecule cannot occupy the same location, lending a decrease in density over the ideal gas. Gases like hydrogen and helium show a more significant contribution from the repulsive force due to finite molecular size, and therefore have slightly lower densities at high pressures. Methane and carbon dioxide show much more significant contributions to their properties from attractive interactions, lending them higher densities than the ideal gas until very high pressures, where the repulsive term dominates (at much higher than 100 bar at 25 °C).

Figure 2. Equilibrium adsorption uptake isotherm of CO2 on high surface area, superactivated carbon MSC-30, at 25 °C.
Applications and Summary
The ideal gas law is such a fundamental equation of the chemical sciences that it has a plethora of uses both in day-to-day laboratory activities as well as in calculations and modeling of even highly complex systems, at least to first approximation. Its applicability is limited only by the approximations inherent to the law itself; at near-ambient pressures and temperatures, where the ideal gas law is well valid for many common gases, it is widely employed in the interpretation of gas-based systems and processes. Two examples of devices that operate on principles, which can be reconciled by use of the ideal gas law, are the gas thermometer and the Stirling engine.
One specific application is in measuring the quantity of gas adsorption (physisorption) on the surface of a solid material. Adsorption is the physical phenomenon whereby gas molecules leave the gas phase and enter into a densified phase near the surface of a solid (or perhaps a liquid) due to attractive intermolecular interactions (dispersion forces) between the solid and the gas. The role of adsorption can be neglected for many bulk materials (such as glass and stainless) steel at ambient conditions, but becomes very important for porous materials with a large accessible surface area, especially at low temperatures.3 The volumetric Sieverts's method and the gravimetric method of quantifying physical adsorption rely on knowing the equation of state of the gas in the system. At low pressures and ambient temperature, the ideal gas law is valid for many gases, and can be used to accurately determine the adsorbed quantity of gas in a similar way as described in the protocol for determining R above. For example, in gravimetric measurements of the buoyancy of a high-surface-area sorbent under conditions where the ideal gas law is in fact valid, the difference between the Δwactual measured and the Δwideal calculated using the ideal equation of state can be attributed to the change in weight of the adsorbed phase. (Equation 10) Equilibrium gas adsorption isotherms can thus be measured by tabulating this deviation, Δwads, as a function of pressure at a fixed temperature (see Figure 2), a standard procedure in the characterization of porous materials.
Δwads = Δwactual - Δwideal (Equation 10)