Overview

The volume occupied by one mole of a substance is its molar volume. The ideal gas law, PV = nRT,  suggests that the volume of a given quantity of gas and the number of moles in a given volume of gas vary with changes in pressure and temperature. At standard temperature and pressure, or STP (273.15 K and 1 atm), one mole of an ideal gas (regardless of its identity) has a volume of about 22.4 L — this is referred to as the standard molar volume.

For example, one mole each of hydrogen, oxygen, argon, or carbon dioxide occupies 22.4 liters at STP. This implies that 0.5 moles of any gas at STP occupies a volume of 11.2 L, and similarly, 2 moles of any gas at STP occupies a volume of 44.8 L.

The ideal gas law is universal, relating the pressure, volume, number of moles, and temperature of a gas regardless of the chemical identity of the gas:

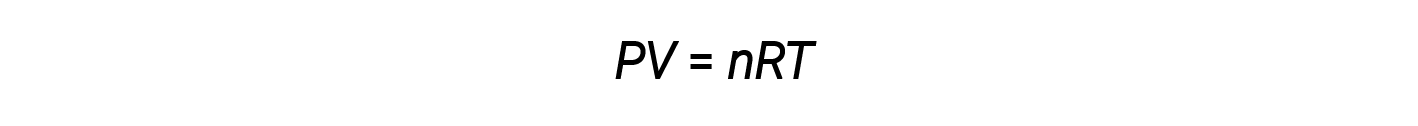
The density d of a gas, on the other hand, is determined by its identity. Density is the ratio of mass over volume. Rearranging the ideal gas equation to isolate V and substituting into the density equation yields:

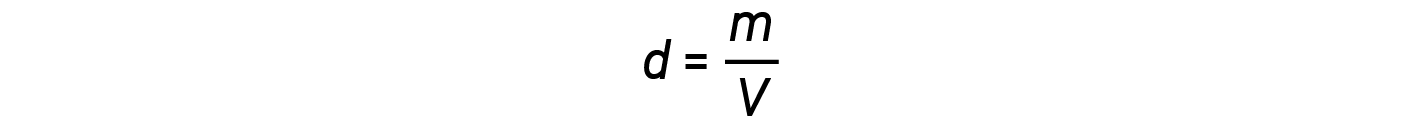
The ratio m/n that is, mass over moles, is the definition of molar mass, M:

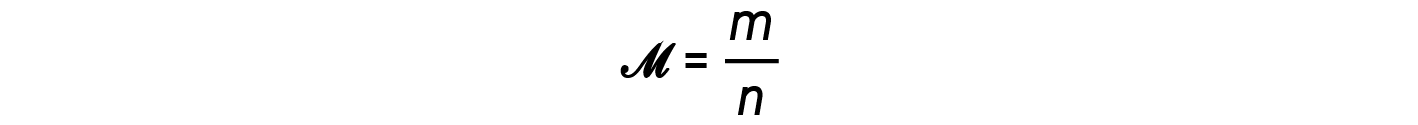
The density equation can then be written as

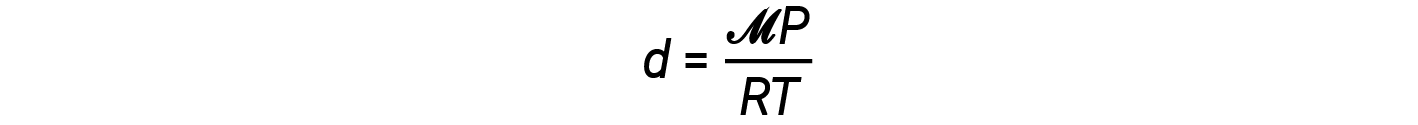
This equation tells us that gas density is directly proportional to the pressure and molar mass, and inversely proportional to the temperature. For example, CO₂ (molar mass = 44 g/mol) is heavier than N₂ (molar mass = 28 g/mol) or O₂ (molar mass = 32 g/mol), and is therefore denser than air. For this reason, CO₂ released from a CO₂ fire extinguisher blankets a fire, preventing O₂ from reaching the combustible material. The phenomenon of the lifting of hot air balloons depends on the relationship that gases of equal molar masses (such as air) have lower densities at higher temperatures, and therefore hot air balloons can float.

This text is adapted from Openstax, Chemistry 2e, Section 9.3: Stoichiometry of Gaseous Substances, Mixtures, and Reactions.

Procedure

All ideal gases conform, in behavior, to a particular relationship between pressure, volume, moles, and temperature as dictated by the ideal gas law.

In this equation, R is the ideal gas constant. Rearranging the equation allows any one of the variables to be calculated as long as the other three are known. 

For example, what is the volume of one mole of an ideal gas under standard temperature and pressure conditions? Abbreviated as STP, these conditions are 0 °C or 273 K and 1 atm. 

Rearranging the equation and substituting in the values for n (1 mole), temperature (273 K), pressure (1 atm), and the ideal gas constant (0.08206 L·atm/mol·K), one mole of an ideal gas occupies a volume of 22.4 liters. This is the molar volume at STP, which is also a good approximation for many common gases.

At higher temperatures and lower pressures, the gas expands and its molar volume is larger than it is at standard conditions. At lower temperatures and higher pressures, the molar volume is smaller.

Another useful quantity of a gas is its density. Recall that the number of moles, n, is equal to the mass of the gas divided by its molar mass. Substituting this relationship into the ideal gas equation, and then rearranging, yields an expression for mass over volume or density. 

From this equation, the density of a gas is directly proportional to its molar mass. This is why helium balloons float away when released outside. The molar mass, and thus density, of helium, is much less than that of air, which is primarily nitrogen and oxygen. 

Also, notice that density and temperature are inversely related. This is observed when piloting a hot air balloon. Turning on the burner heats up the air molecules within the balloon and they move faster. 

The pressure in the balloon increases, but the balloon is designed so that some of the air escapes. This makes the air in the balloon less dense than the surrounding air. Because of this difference in density, the balloon ascends.

Conversely, turning off the burner and opening the vent, allows the warm to escape. As the balloon contracts, outside air enters, increasing the density in the balloon to that of the surroundings. Then, because of the weight in the basket, the balloon descends.

The equation, when rearranged, also allows us to calculate the molar mass of an unknown gas.

Suppose an unknown gas with a mass of 12.5 grams occupies a volume of 6.08 liters and exerts a pressure of 1.2 atm at 40.0 °C.

The density of the gas is known from the given mass and volume. Then, the temperature in degree Celsius is converted to units of kelvin and substituted into the equation along with the values for pressure and the gas constant.

Solving for M yields a molar mass of 44 g/mol. Therefore, carbon dioxide is the unknown gas.